Equivalent Fractions Reduce to the Same Simple Form

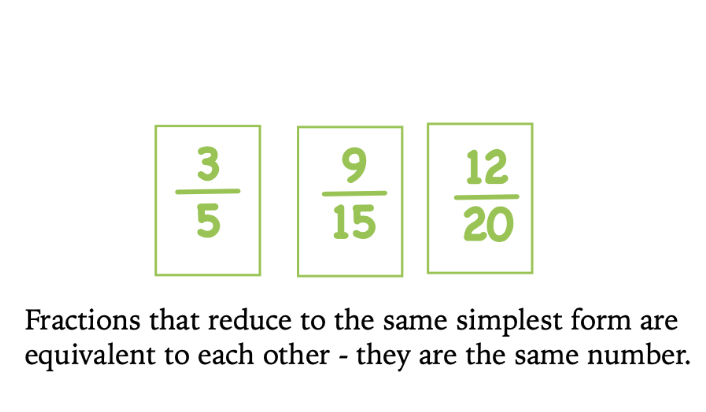
What are equivalent fractions?

Equivalent fractions:

• share the same location on the number line.
• ARE exactly the same number, written in a different form.
• can be used interchangeably to solve equations.
• take up the same amount of area.
• reduce to the same simple form.

Reducing numbers to their simplest form is complex.

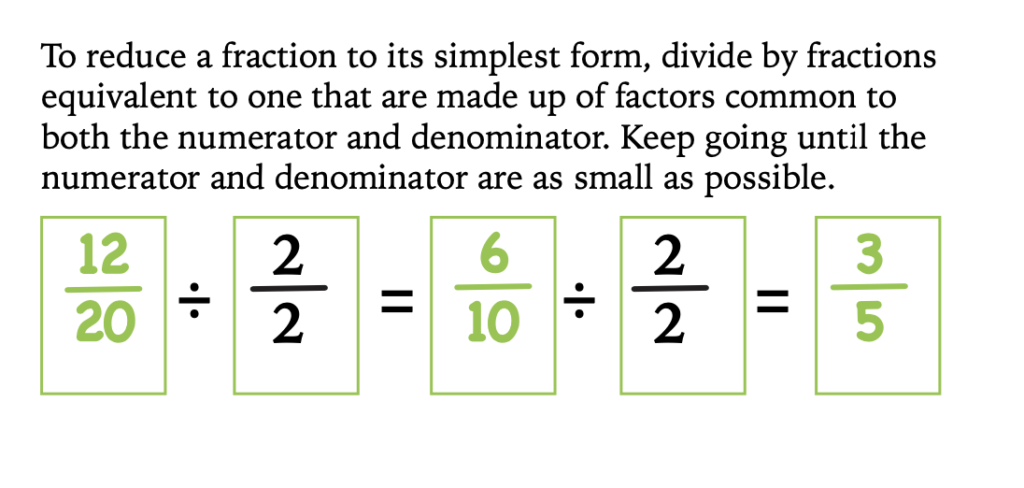
Let your students get plenty of practice reducing fractions to their simplest form before you ask them to use this method as a test of equivalence.

When they start reducing fractions, you students will not be able to find the largest common factor the first time. Give them time to get used to the process of reducing fractions.

Ask questions to guide them such as: How do you know when the fraction is in its simplest form? How did you decide to divide by 2/2 in this step? What role does multiplication play in reducing fractions to their simplest form?

Fractions are in their simplest form when the numerator and denominator no longer have any common factors. When both the numerator and denominator are even numbers, you know that they have a factor of 2 in common. Equivalent fractions are created by multiplying the simplest form by fractions equivalent to one ~ division is the opposite of multiplication so dividing by fractions equivalent to one will not change the number and will also shrink the numerator and denominator to their smallest possible pair of digits which can express that number.

Remind your students that just as multiplying by one does not change a number, dividing numbers by one does not change the number either. Some students might need to review the work they did previously in order to understand how the Multiplicative Identity Property of One helps them reduce fractions to their simplest form. Multiplying or dividing by one does not change a number’s identity.

Students need many hours of thoughtful practice before they will begin to make sense of what simplest form means.

With thoughtful practice and class discussions on how to make the process more efficient, your students will eventually be ready to learn how to reduce fractions to their simplest form in the fastest way possible.

Calculating with fractions is messy and complicated. Let your students dive in and embrace the mess and then simplify their answers. After a while, have a class discussion on when to simplify fractions in an equation. Will it affect the answer if you simplify fractions along the way or if you wait until the end of process and reduce the answer? If your students can’t convince you that it doesn’t matter at all, then challenge them to try both ways on the same equations. Let them keep solving equations both ways until they know for a certain fact that it makes no difference when fractions are simplified during the process of solving equations. 

Introduce your students to prime numbers gently. They have been trained to look for and think about multiples of numbers. By their definition prime numbers are not multiples of any numbers other than themselves and one. Students need time to switch their thinking from looking for multiples, to looking between the multiples.

Spend plenty of class time exploring prime numbers. Provide many different kinds of opportunities for your students to figure out the prime factors of numbers before you ask them to use this as a method for reducing equivalent fractions to their simplest form.

Make this task easier by having students work in pairs or small groups to get started. Let them talk about the process of reducing fractions to their simplest forms during class discussions.

After your students are consistently able to reduce fractions to their simplest forms using their own haphazard methods, and after they have had lots of experience with prime numbers, offer to show them a cool shortcut that will help them reduce fractions quickly. 

Since they have personally wrestled with the issue, and the understand the concepts, they will be ready to appreciate using prime factors to find a fraction’s simplest form.

Eventually, your students will be able to reduce a pair of fractions to their simplest forms in order to test whether or not the numbers are equivalent.